UM Directory

Courses Taught

Current courses (Spring 2023):

STAT 216: Introduction to Statistics

Past courses

M132 Number and operations for K-8 teachers (F 2015; S 2016; F 2016; S 2017; F 2017; S 2018; S 2019; S 2020; F 2020)

M429 History and nature of mathematics (S 2017)

M500 Current mathematics curricula (S 2016; S 2017; S 2019)

M510 Problem solving for teachers (F 2017; F 2019)

M572 Algebra for teachers (Sum 2016; Sum 2018; Sum 2020; Sum 2022) 

M574 Probability and statistics for teachers (Sum 2017; Sum 2021)

M595 Philosophy of education and mathematics education (S 2022)

M595 Advanced research methods in math education (F 2022)

M596 Qualitative research methods (S 2018)

M596 Teaching and learning in Calculus (S 2019)

M602 Teaching college math (F 2016; S 2020)

STAT 216 Introduction to statistics (F 2020; S 2021; F 2021; S 2022; F 2022; S 2023)

Seminars

F 2017: Alternative forms of knowing in mathematics

F 2019: Rehumanizing mathematics for Black, Indigenous, and Latinx Students

F 2021: The theory of objectification 

Websites

Resources for Teaching college math

Resources for Teaching mathematics through problem solving

OER Textbooks

Statistical thinking: A simulation approach to modeling uncertainty (Modified from Zieffler & Catalysts for Change, 2019) 

• Textbook
• Classroom activities

Teaching Experience

2021-Present: Associate professor of Mathematics Education, Department of Mathematical Sciences, University of Montana

2015-2021: Assistant professor of Mathematics Education, Department of Mathematical Sciences, University of Montana

2013-2015: Instructor, School of Education, University of Colorado

2006-2012: Math teacher, Centaurus High School (Lafayette CO)

Research Interests

My research draws on two traditions in mathematics education and the learning sciences: Realistic Mathematics Education (RME) and cultural-historical perspectives on learning. RME starts with the premise that mathematics is, first and foremost, an activity, the human activity of structuring the world. Cultural-historical perspectives on learning are also concerned with human activity. From a cultural-historical perspective, the primary features of human activity are (a) that it is productive, and (b) that it is intertwined with the products of prior activity. Thus my research examines the following big question:

“what gets produced as people engage in mathematical activity?”

In short, my answer is, activity, artifacts, community, and identity are all “productively intertwined,” with each producing and being produced by, the others:

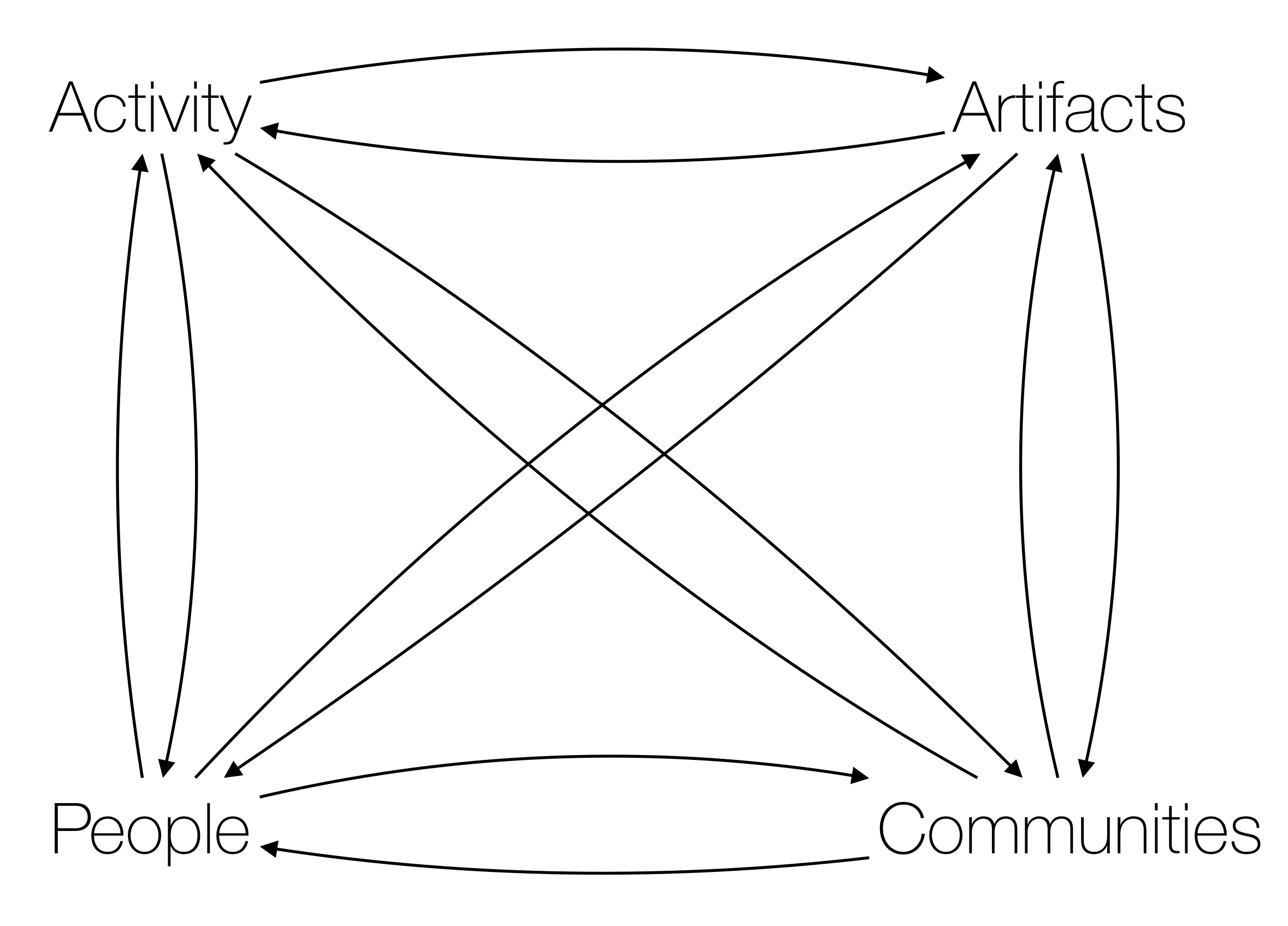
I view all aspects of this mutual production to be at play in all mathematical activity. However, I find it productive to focus different strands of my research on particular aspects, as described in the "Projects" section, below.

Projects

Strand 1: The productive intertwinement of activity, artifacts, and identity. 

As humans engage in activity, we produce and accumulate “partial solutions to frequently encountered problems” (Hutchins, 1995, pp. 354–355). These partial solutions are called “cultural artifacts.” Mathematical artifacts include the “content” of mathematics, including concepts, models, tools, strategies, representations, algorithms, and notation systems. Artifacts serve to make the accomplishments of prior activity available in the present, and human activity always incorporates artifacts. Moreover, as humans act with artifacts, the artifacts “act back,” working to produce the human actors in particular ways. For example, mathematical artifacts help to produce particular kinds of mathematical identities, including identities as “a math person” or “not a math person.”

Taken together, this leads me to study the productive intertwinement of activity, artifacts, and identity:

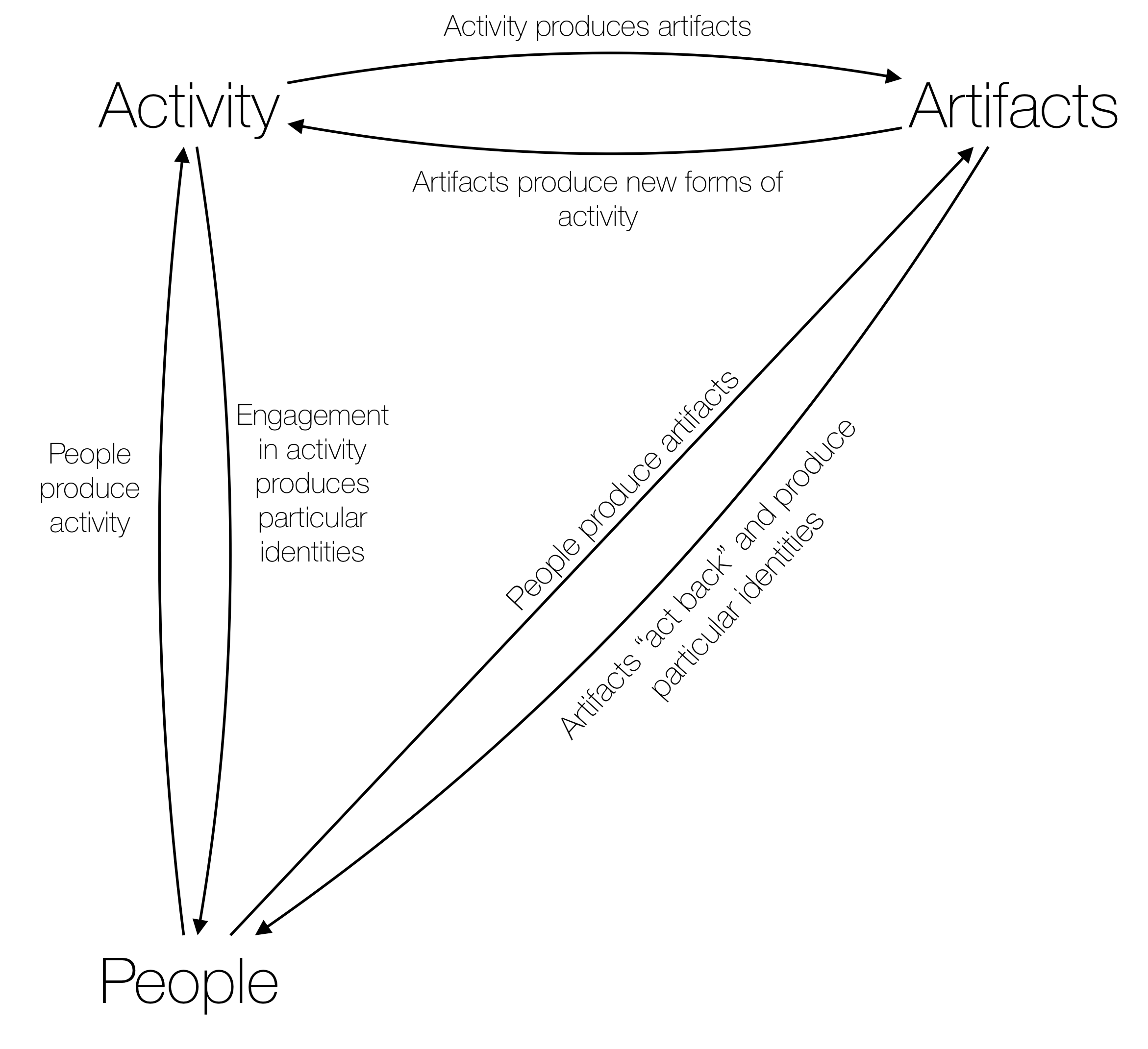
This strand of research is prominent in the following research projects: 

• Student learning in high school algebra. In a series of design-based research studies, my colleagues and I studied how mathematical artifacts were reinvented and made meaningful in activity in a high school algebra classroom, and the implications for students’ identities.
• Teacher learning about classroom assessment. In a research-practice partnership, our research team collaborated with a group of teachers to design classroom assessment systems based on learning trajectories. This involved the intentional production of particular artifacts, and research into how these and other institutional artifacts mediated teachers’ activity.
• Infrastructures and identities in engineering school: In this field-based ethnography, my colleagues and I studied the organizational infrastructure of a prominent engineering school, and how that infrastructure worked to produce students as particular kinds of people.

Strand 2: The productive intertwinement of activity, identity, and community

Above, I described how I seek to understand mathematical activity as a cultural endeavor. I also seek to understand activity as a social endeavor. To do so, I take the perspective that in activity, humans relate to other humans and thus it is useful to conceptualize activity as a joint enterprise. As people engage in joint activity, they form communities. These communities are produced by their members and by the joint activity that binds the members together. In turn, activities derive meaning and people develop identities by virtue of their situatedness within communities. 

Taken together, this leads me to study the ways in which mathematical activities, communities, and identities are productively intertwined:

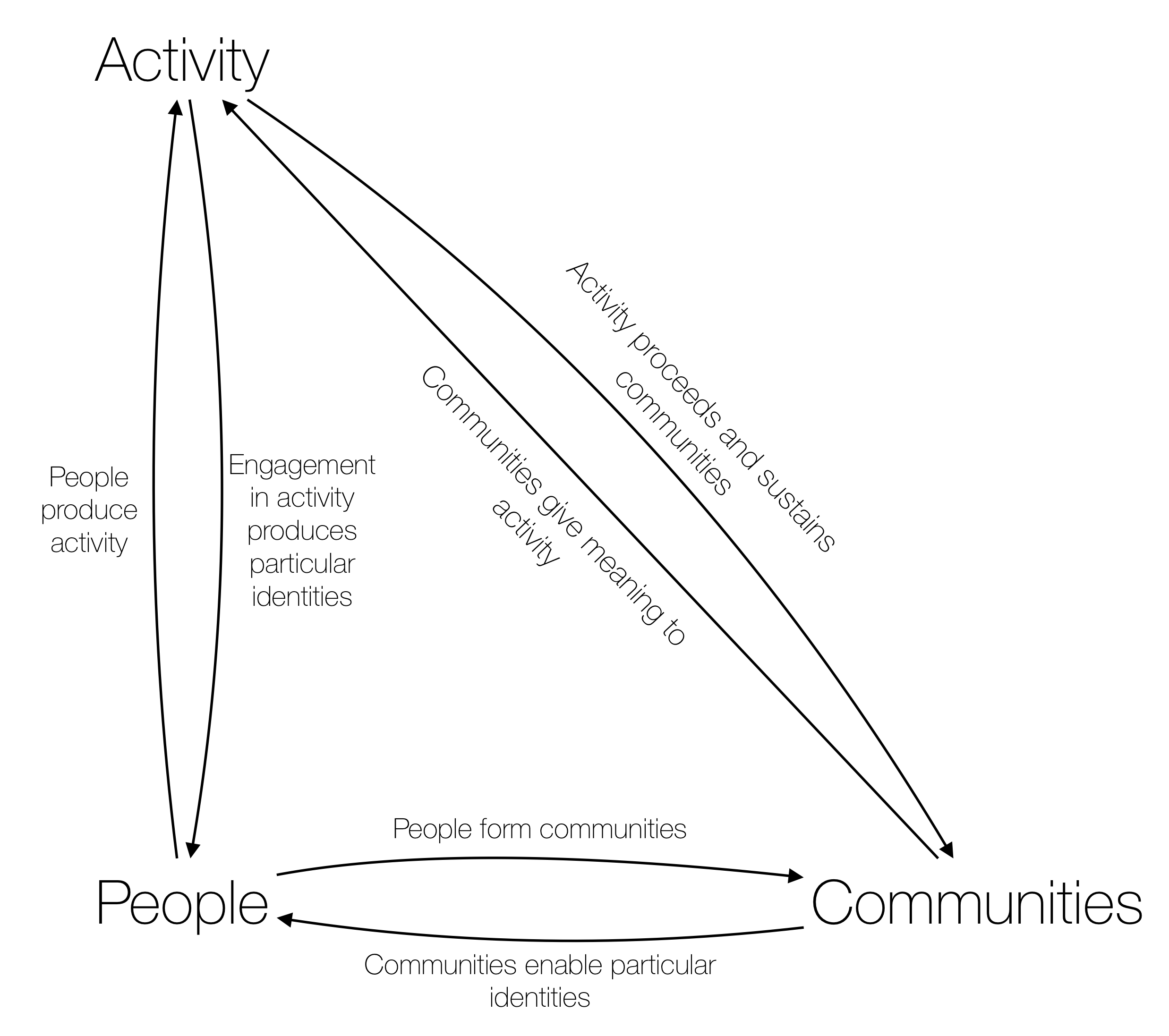
This strand of research is prominent in the following research projects: 

• Community and identity in Math Teachers’ Circles. In this field-based ethnography, my colleagues and I study the longitudinal development of communities of math teachers who gather together to engage in mathematical activity. We are interested in the kinds of activities the groups engage in, and the implications for the development of community and identity. This project is a multi-institution collaboration.
• Montana Models: In this study we collaborate with youth in rural communities and American Indian nations to address a community-based project that is of interest to youth, using both local practices and mathematical practices. This involves the following activities: (1) We use ethnographic methods to study the local problem solving practices in the community. (2) We use design-based research to design and study summer camps for youth that act as hybrid spaces to bring mathematical activity and local problem solving practices into contact. (3) We work with the youth to identify a community project and to address that project using both local and mathematical practices. This project is a multi-institution collaboration.

Field of Study

Mathematics Education

Publications

Peer reviewed publications

Peck, F.A., Renga, I.P., Wu, K., & Erickson, D. (2022). The durability and invisibility of practice fields: Insights from math teachers doing math. Cognition and Instruction, 40(3), 385–412. https://doi.org/10.1080/07370008.2021.1983577

Peck, F.A. (2022). Finding learning in “off-task” behavior: Artifacts, agency, and second stimuli. For the Learning of Mathematics, 42(1), 31–34.

Peck, F.A., Johnson, R., Briggs, D.C., & Alzen, J. (2021). Toward learning trajectory-based instruction: A framework of conceptions of learning and assessment. School Science and Mathematics, 121, 357–368. https://doi.org/10.1111/ssm.12489

Peck, F.A. (2021). Towards anti-deficit education in undergraduate mathematics education: How deficit perspectives work to structure inequality and what can be done about it. PRIMUS, 31(9), 940–961.https://doi.org/10.1080/10511970.2020.1781721 [full text]

Renga, I. P., Peck, F. A., Wu, K., & Erickson, D. (2020). Fueling teachers’ passion and purpose. Educational Leadership, 78(4), 68–71. [full text]

Peck, F.A. (2020). Beyond rise over run: A learning trajectory for slope. Journal for Research in Mathematics Education, 51(4), 433–467. https://doi.org/doi:10.5951/jresematheduc-2020-0045 [full text]

Renga, I.P., Peck, F.A., Feliciano-Semidei, R., Erickson, D. & Wu, K. (2020). Doing math and talking school: Professional talk as producing hybridity in teacher identity and community. Linguistics and Education, 55, 100766. https://doi.org/10.1016/j.linged.2019.100766 [full text]

Peck, F.A. (2018). Rejecting Platonism: Recovering humanity in mathematics education. Education Sciences, 8(43). https://doi.org/10.3390/educsci8020043 [full text]

Peck, F.A. & Sriraman, B. (2017). Breaking the constraints of modernist psychologizing: Mathematics education flirts with the postmodern. Interchange, 48, 351-362. https://doi.org/10.1007/s10780-017-9306-1 [full text]

Peck, F.A., Erickson, D., Feliciano-Semidei, R., Renga, I. Roscoe, M., & Wu, K. (2017, October). Negotiating the essential tension of teacher communities in a statewide Math Teachers’ Circle. Proceedings of the 39th annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education. Indianapolis, IN. [full text]

Peck, F.A. & Matassa M. (2016). Reinventing fractions and division as they are used in algebra: The power of preformal productions. Educational Studies in Mathematics, 92, 2, 245-278. https://doi.org/10.1007/s10649-016-9690-y [full text]

Peck, F. A., O’Connor, K., Cafarella, J., & McWilliams, J. (2016, October). How borders produce persons: The case of calculus in engineering school. In M. B. Wood, E. E. Turner, M. Civil, & J. A. Eli (Eds.), Proceedings of the 38th annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education (pp. 1079–1086). Tucson, AZ: The University of Arizona. [full text]

O’Connor, K., Peck, F.A., McWilliams, J. & Cafarella, J. (2016, June). Working in the weeds: How do instructors sort engineering students from non-engineering students in a first year pre-calculus course? Proceedings of the 2016 American Society for Engineering Education Annual Conference and Exposition, New Orleans, LA. [full text]

Briggs, D. and Peck, F.A. (2015). Rejoinder to commentaries on Using learning progressions to design vertical scales that support coherent inferences about student growth. Measurement: Interdisciplinary Research and Perspectives 13, 3-4, 206-218. https://doi.org/10.1080/15366367.2015.1104113 [full text]

Briggs, D. and Peck, F.A. (2015). Using learning progressions to design vertical scales that support coherent inferences about student growth. Measurement: Interdisciplinary Research and Perspectives 13, 2, 75-99. https://doi.org/10.1080/15366367.2015.1042814 [full text]

O’Conner, K., Peck, F.A., and Cafarella, J. (2015) Struggling for legitimacy: Trajectories of membership and naturalization in the sorting of engineering students. Mind, Culture, and Activity 22, 2, 168-183. https://doi.org/10.1080/10749039.2015.1025146 [full text]

O’Connor, K., Peck, F.A., & Cafarella, J. (2015). Constructing “calculus readiness”: Struggling for legitimacy in a diversity-promoting undergraduate engineering program.  Proceedings of the 2015 American Society for Engineering Education Annual Conference and Exposition, Seattle, WA: ASEE. 26.397.1-26.397.17 [full text]

O’Connor, K., McWilliams, J., Peck, F.A., & Cafarella, J. (2015). Ideologies of depoliticization in engineering education: A Mediated Discourse Analysis of student presentations in a first-year projects course  Proceedings of the 2015 American Society for Engineering Education Annual Conference and Exposition, Seattle, WA: ASEE. 26.880.1-26.880.17 [full text]

Matassa, M. & Peck, F.A. (2012). Rise over run or rate of change? Exploring and expanding student understanding of slope in Algebra I. Proceedings of the 12th International Congress on Mathematics Education. Seoul, Korea. 7440-7445.

Book chapters

Webb, D.C., & Peck, F.A. (2020). From tinkering to practice — The role of teachers in the application of Realistic Mathematics Education principles in the United States. In M. van den Heuvel-Panhuizen (Ed.), International reflections on the Netherlands didactics of mathematics: Visions on and experiences with Realistic Mathematics Education (pp. 21–39). Springer. [full text]

Honors / Awards

Helen and Winston Cox Educational Excellence Award, Univeristy of Montana

William Stannard Award for the Teaching of Undergraduate Mathematics in Montana, Montana State University.

Best Should Teach award, University of Colorado

Chancellor's Fellow, University of Colorado